ЁЯУв Too many exams? DonтАЩt know which one suits you best? Book Your Free Expert ЁЯСЙ call Now!

    Question

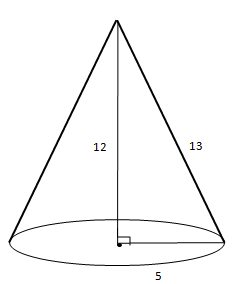
    The height of a solid cylinder is 30 cm and the diameter

    of its base is 10 cm. Two identical conical holes each of radius 5 cm and height 12 cm are drilled out. What is the surface area (in cm²) of the remaining solid?
    A 430 ╧А Correct Answer Incorrect Answer
    B 230 ╧А Correct Answer Incorrect Answer
    C 330 ╧А Correct Answer Incorrect Answer
    D 120 ╧А Correct Answer Incorrect Answer

    Solution

    According to question: Height of cylinder = 30 cm Radius of cylinder = 5 cm Height of cone = 12 cm Radius of cone = 5 cm We know that, l2 = h2 + r2 ⇒ l2 = 122 + 52 ⇒ l2 = 144 + 25 ⇒ l = 13 cm The surface area of the remaining figure = surface area of cylinder + 2 × surface area of the cone ⇒ 2πrh + 2πrl ⇒ 2πr(h + l) ⇒ 2π × 5(30 + 13) ⇒ 430π ∴ The surface area of the remaining solid is 430π.

    Practice Next
    More Quant Miscellaneous Questions

    Relevant for Exams:

    ask-question