Question
If the areas of two similar triangles are in the ratio
196 : 625,what would be the ratio of the corresponding sides?Solution
ATQ, The ratio of the areas of two similar triangles is equal to the square of the ratio of their corresponding side lengths. Let the ratio of the corresponding side lengths be a:b. Then, the ratio of their areas would be (a2) : (b2). Given that the ratio of the areas is 196:625, we can set up the equation: (a2) : (b2) = 196 : 625 To find the ratio of the corresponding side lengths, we can take the square root of both sides of the equation: √[(a2) : (b2)] = √(196 : 625) Simplifying, (a/b) = √(196/625) (a/b) = 14/25 Therefore, the ratio of the corresponding side lengths is 14:25.
Which of the following languages is/are spoken in Meghalaya?
1) Kokborok
2) Garo
3) Khasi
Consider the following information:
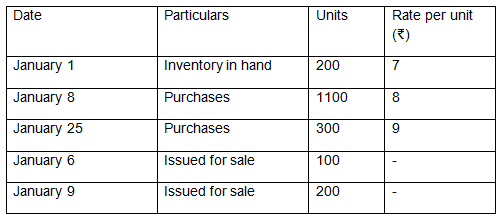
Which one of th...
Which of the following awards was received by Pandit Birju Maharaj in1986 ?
Kharavela was an important ruler of which dynasty?
How many companies were amalgamated and grouped into four major insurance companies?
As on July 2020, employees whose monthly wages are ______ or below are covered under the ESI Act.
Who called Mahatma Gandhi’s ways as Political Blackmail?
In which of the following Sessions of the IOC Executive Board Meeting was Brisbane voted to host the 2032 Olympics and Paralympics?
In MS-Word 2016, the ‘Aa' feature within the Font group of the Home tab represents the ________ option.
In which area was the origin of Kalighat art?
Relevant for Exams:


