Question
A sniper on the parapet of a 50 feet high building was
aiming at a target. The angle of depression that his rifle made was 45 °. How far away from the building was his target?Solution
 Let θ be the angle of depression of the point on the ground as seen from the top of a tower, here θ = 45° Let AC be the height of the tower, here AC = 50 feet. Let the distance of the point on the ground from the foot of the tower, AB = x feet. Here, tan θ = AC/AB ⇒ tan 45° = 50/x ⇒ 1 = 50/x ⇒ x = 50 feet
Let θ be the angle of depression of the point on the ground as seen from the top of a tower, here θ = 45° Let AC be the height of the tower, here AC = 50 feet. Let the distance of the point on the ground from the foot of the tower, AB = x feet. Here, tan θ = AC/AB ⇒ tan 45° = 50/x ⇒ 1 = 50/x ⇒ x = 50 feet
Arvind Panagariya is the chairman of which finance commission?
Which component of the Vikram 1 launch vehicle was successfully test-fired in March 2024 by Skyroot Aerospace?
The recently signed Indo-Pacific Economic Framework (IPEF) focuses on collaboration between which countries?
As per the Reserve Bank of India (RBI) bi-annual Financial Stability Report (FSR) for June 2024 , what is the approximate contribution (%) of PSU Bank...
Which hormone regulates the blood sugar level in the body?
Consider the following information:
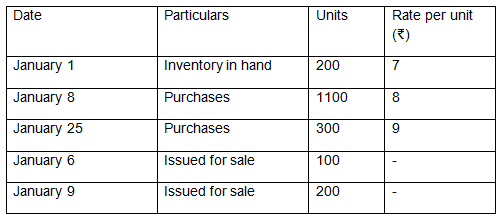
Which one of th...
Project Nexus is a multinational initiative for linking real-time payment systems. Which of the following countries is NOT a part of Project Nexus?
'Ali-Aye-Ligang', a spring festival, is associated with which state?
______ became the first Indian to win the under-18 title in the Orange Bowl tournament in 2008.
Mangrove vegetation in India is most extensive in ________.
Relevant for Exams:


