Question
Four of the five among the following are similar in such
a way to form a group. Which one of the following doesn’t belong to the group? Study the following information to answer the given questions : Six persons A, B, C, D, E and F are sitting in a triangular table, such that three of them sitting at the corners of the table and three are sitting at middle of the each side. The persons who are sitting at the corners are facing outside the centre and the persons who are sitting at middle of the sides are facing the centre of the table. Each person likes different cold-drink namely – Coca-Cola, Thumsup, Pepsi, Sprite, Miranda and Maaza. All the information is not necessary to be in the same order. (i) E likes Thumsup and sits second to right of the one who likes Miranda. D doesn’t face opposite to the centre. C sits immediate left of the one who likes Sprite. (ii) B and D are not immediate neighbours. The one who likes Coca-Cola sits second to the right of B. F doesn’t like Sprite. Two people sit between the one who likes Sprite and the one who likes Pepsi. (iii) Two people sit between A and the one who likes Maaza. The one who likes Pepsi sits second to the right of the one who likes Maaza.Solution
From (iii), we will have two cases: Case 1. If the one who like Maaza sits at place no.1, then the one who likes Pepsi sits at place no.5. A will sit at place no. 4. From (ii), the one who likes Sprite sits at place no.2. From (i), C sits at place no.1. E and the one who like Miranda sit at place no. 4 and 6 respectively. D will sit at place no. 2. B will sit at place no. 5. Only person left for place no. 3 is F, who likes Coca-Cola. 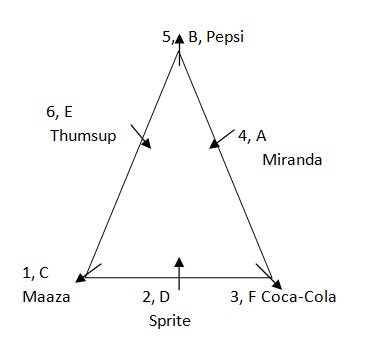 Case 2. If the one who like Maaza sits at place no.2, then the one who likes Pepsi sits at place no. 4. A will sit at place no. 5. From (ii), the one who likes Sprite sits at place no.1. From (i), C sits at place no.2. E and the one who like Mirinda sit at place no. 3 and 5 respectively. B and the one who likes Coca-Cola sit at place no. 4 and 6 respectively. This case will get discarded as F does not like Sprite.
Case 2. If the one who like Maaza sits at place no.2, then the one who likes Pepsi sits at place no. 4. A will sit at place no. 5. From (ii), the one who likes Sprite sits at place no.1. From (i), C sits at place no.2. E and the one who like Mirinda sit at place no. 3 and 5 respectively. B and the one who likes Coca-Cola sit at place no. 4 and 6 respectively. This case will get discarded as F does not like Sprite.  Final arrangement as shown below:
Final arrangement as shown below: 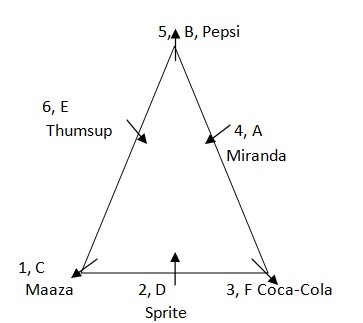
The average weight of (n + 1) girls is 50 kg, and the average weight of (n - 3) boys is 70 kg. If the overall average weight is 62 kg, how many boys are...
- The average weight of 50 workers in a factory is 70 kg. Two workers weighing 75 kg and 68 kg leave the job and are replaced by two others weighing 72 kg an...
A football player scored 400 points in the first eight games of the season. Find the average points he must score in the next two games so that his aver...
Average run scored by a batsman in first ‘x’ matches is 50. In next 16 matches he scored runs with an average of 70 so that his overall average incr...
Sum of ages of 55 team members is 1220 years. The average age of 20 of them is 19 years and the average age of another 15 of them is 20 years. Find the ...
- In a company team, if 3 employees each weighing 65 kg resign, the average weight of the remaining team members drops by 1 kg. But if 4 employees with an av...
The average of n numbers is 66. If each of 54% of the numbers is increased by 13 and each of the remaining numbers is decreased by 12, then the n...
- Rs. 4200 was distributed among 'P', 'Q', 'R' and 'S' in the ratio 2:5:4:3 respectively. Later, 'Q' gave an amount equal to 80% of the average amount receiv...
Average run scored by a batsman in first ‘x’ matches is 44. In next 8 matches he scored runs with an average of 80 so that his overal...
The average marks of 'm' students in a math test is (m + 5) and the average marks of 30 girls is (2m - 10). If the average marks of all students togethe...
Relevant for Exams:


