Question
If cos(2θ) = 1 - 2sin²(θ) and tan(θ) + cot(θ) = k,
find the value of k when sin(θ) = 3/5.Solution
We are given sin(θ) = 3/5. Now, find cos(θ): Using the Pythagorean identity: cos(θ) = √(1 - sin²(θ)) = √(1 - (3/5)²) = √(1 - 9/25) = √(16/25) = 4/5. tan(θ) = sin(θ) / cos(θ) = (3/5) / (4/5) = 3/4. cot(θ) = 1 / tan(θ) = 4/3. tan(θ) + cot(θ) = (3/4) + (4/3). = 9/12 + 16/12 = 25/12. Thus, the value of k is 25/12
(tan 5x - tan 3x - tan 2x) = ?
cot 13˚
 cot 14˚ ...
cot 14˚ ...A tower is 60 meters high. From a point on the ground, the angle of elevation of the top of the tower is 30°. Find the distance of the point from the b...
If sec 2A = sin2 60o + sec 60o - cos2 30 o , then find the value of (√3tan A + cot ...
If sin θ = 3/5 and cos θ = 4/5, find the value of (2tan² θ + 3cot² θ).
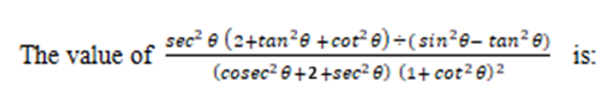
Take θ = 450
- If X = 10°, find the value of:
cos²(3X) + sin²(6X) - tan²(3X) - If cos2B = sin(1.5B - 36 o ) , then find the measure of 'B'.
- Calculate the value of sin120 o + cos180 o + tan225 o .
ABC is an isosceles triangle where AB = AC which is circumscribed about a circle. If P is the point where the circle touches the side BC, then which of ...


