New RBI Grade B 2025 Notification will come Soon! Enroll Here

    Question

    There are two houses of the same height on both sides of

    a 30-meter wide road. From a point on the road, elevation angles of the houses are 30° and 60° respectively. Find the height of the houses.
    A 10.8 meter Correct Answer Incorrect Answer
    B 12.9 meter Correct Answer Incorrect Answer
    C 25.2 meter Correct Answer Incorrect Answer
    D 10 meter Correct Answer Incorrect Answer

    Solution

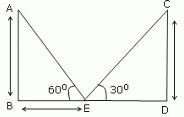    Let y be the height of the houses, here AB = CD = y meter. Let x be the distance between the point on the road and the house making an elevation angle of 60°, here BE = x meter. Then, (30 - x) is the distance between the point on the road from the house making an elevation angle 30°, here DE = (30 - x) meter. Now, tan 60° = AB/BE ⇒ √3 = y/x ⇒ x = y/√3 ...(i) Also, tan 30° = CD/DE ⇒ 1/√3 = y/(30 - x) ⇒ √3y = 30 - x ⇒ √3y = 30 - y/√3 [from equation i] ⇒ 3y = 30√3 - y ⇒ 4y = 30√3 ⇒ y = 30√3/4 ≈ 12.9 meter

    Practice Next

    Relevant for Exams: