Question
AB is the diameter of a circle centered at O. A tangent
drawn at point C intersects the extension of AB at point D. If ∠CAB = 38°, find the value of ∠CDB.Solution
ATQ,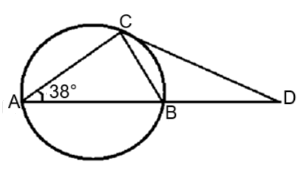
Angle made by the diameter of a circle on the arc of the circle is 90°, i.e. ∠ACB = 90°.
Sum of all angles in a triangle is 180°.
So, ∠CAB + ∠CBA + ∠ACB = 180°
Or, 38° + ∠CBA + 90° = 180°
Or, ∠CBA = 180° - 128°
Or, ∠CBA = 52°
Now, ∠CBA + ∠CDB = 180°
(Linear pair of angles)
So, ∠CDB = 180 - 52 = 128°
The class NP-complete includes problems that are:
Which of the following is NOT a method for handling deadlocks?
Which of the following statements is true regarding the class NP?
What is a deadlock in a computer system?
Which famous sorting algorithm uses the divide-and-conquer strategy?
Which data structure in a compiler is used for managing information about variables and their attributes?
Which command will help you to find the time of how long the system is being running?
In which case higher bandwidth can be achieved
What is an algorithm?
In the context of ADTs, what does "encapsulation" refer to?
Relevant for Exams:
