New Enroll here to Join NaBFID Analyst Into Class on May 6

    Question

    An edge of a variable cube is increasing at the rate of

    2 cm/s. How fast is the volume of the cube increasing when the edge is 12 cm long?
    A 804 cm^3/s Correct Answer Incorrect Answer
    B 764 cm^3/s Correct Answer Incorrect Answer
    C 864 cm^3/s Correct Answer Incorrect Answer
    D 664 cm^3/s Correct Answer Incorrect Answer

    Solution

    Let x be the length of a side and V be the volume of the cube. Then, v = x3. dV/dt = 3x2 × dx/dt It is given that dx/dt = 2 cm/s dv/dt = 3x2(2) = 6x2 Thus, when x = 12 cm dv/dt = 6 (12)2 = 864 cm3/s

    Practice Next

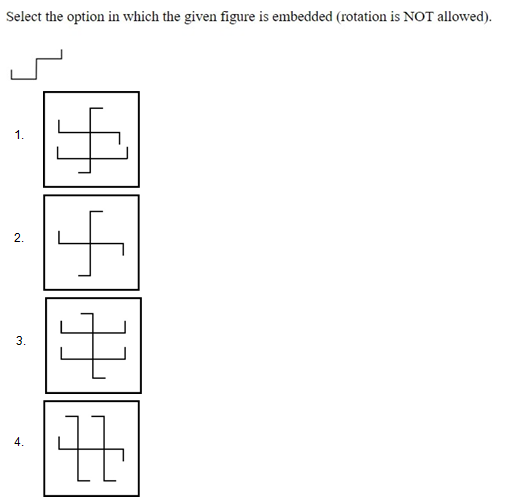
    Relevant for Exams: